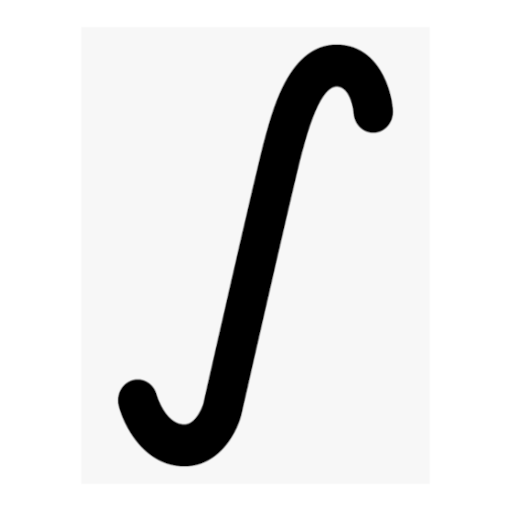
Find x for xxxxx^… = 2
First off, why did this post get downvoted?
Answer alert
The answer is sqrt(2), because sqrt(2)2 = 2, and you build the infinite exponential sequence by taking x_n = xx_(n-1).
yup there u go, btw can u please spoiler it so its harder for people to accidentally see it
Now try xxx^… = 4. And then tell me why it’s not a logical crisis that you get exactly the same answer when you solve xxx^… = 2 instead.
The reason is that xx… doesn’t converge to anything greater than e.
Here’s what happens with sqrt(2) as x with a 12 large power tower (WolframAlpha doesn’t like power towers with roots):
Here’s what happens with e(1/e), the max value for x with a 500,000 large power tower:
(Note: I was unable to enter that value so I approximated it. The exact result should be e)
Here’s what happens with the value above +0.00001 and a mere 1023 large power tower:
You can read the reasons here, I couldn’t follow for very much but maybe you can get something out of it:
What is weird is that xxxx… does have multiple results as for e.g. x=√2 it can yield 2, 4, and possibly more. Maybe it is due to the concept of infinity that we cannot really grasp.
spoiler
Allow y = xxx^…
Notice the exponent tower contains itself, such that:
y = x^y
Raise both sides to 1/y power:
y^(1/y) = x^(y/y)
y^(1/y) = xFor y = 2,
2^(1/2) = x
Aka:
x = √2